Introduction
Partaking in an introduction to calculus lesson is essential because the emergence of technology has increased the demand for mathematically skilled people. As a result, a higher burden has been placed on mathematics departments to train a more diverse and extensive pool of students on campus. Many factors contribute to the success rate of the lesson, including the effectiveness of placement examinations, quality of teaching, and learners’ prior experiences with the subject. For instructors to ensure students have acquired all necessary skills in the class, it is required to conduct task analysis (Brown & Green, 2020). The process involves identifying and explicitly teaching students how to perform each step involved in calculating derivatives of functions and integrating. Task analysis helps educators select the most vital task that aligns with the lesson’s goal and most benefits learners (Khoshaim & Aiadi, 2018) This assessment highlights the instruction content needed for an introduction to calculus lesson and the order in which this information will be taught. Additionally, the essay discusses the depth of learning based on the previously conducted needs analysis.
Broad Level Task to be Completed
The needs analysis of the lesson revealed that many students fail introduction to calculus examinations because they do not understand the arithmetic and algebra associated with concepts of the class. Most learners know the calculus concepts and formulas, such as the chain rule, product rule, and quotient rule. However, they cannot critically think and apply the correct principle to some questions in an exam (Gordon, 2022). The primary reason for this problem is that students do not have adequate critical thinking skills to understand and apply concepts that underlie calculus algebraic expressions. Most learners often struggle with differentiating and integrating problems whose roots can be grounded in challenges related to critical thinking. Calculus has many formulas and concepts that students can apply when solving functions-choosing the wrong algorithm makes the final answer wrong (Hitt & Dufour, 2021). The most common mistake made by learners is copying procedures used in the examples in the textbook without considering other competencies used. Therefore, the broad-level task that should be completed through this lesson is equipping learners with the necessary cognitive skills to integrate and differentiate functions appropriately. As a result, students will overcome difficulties and comprehend conceptual knowledge better, performing well in subsequent calculus lessons.
Overview of Analysis of Task Aspects
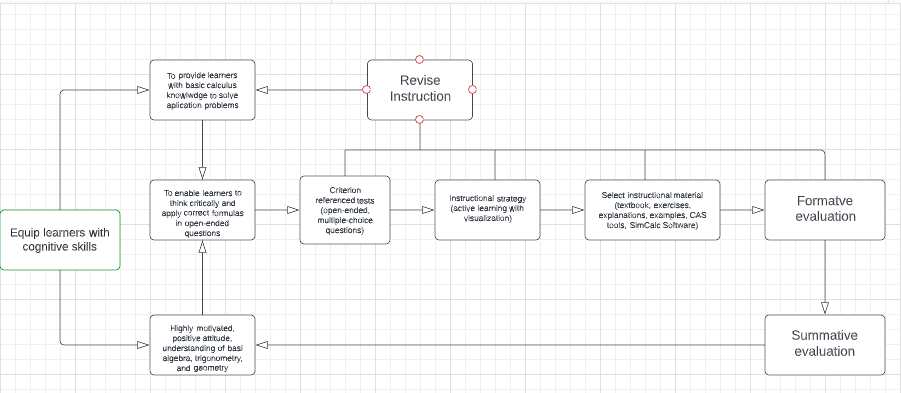
The aspects of the aforementioned task will be analyzed through a series of steps, which ensure all critical components of the lesson are taught. The evaluation is based on Dick and Carey’s instructional model (Almazyad & Alqarawy, 2020). The initial step in breaking down this task is identifying the lesson’s goal. For example, the lesson’s objective in this case would be to equip students with necessary skills to compute the integrals, limits, and derivatives of functions. Another purpose would be to enable students to identify the correct principles and formulas to solve applied calculus problems. In defining the purpose of a class, educators should state what students will be able to do at the end of the class (Brown & Green, 2020). The introduction to the calculus course purposes to provide learners with basic integral and differential calculus knowledge. Consequently, this will enable them to solve application problems that need such knowledge.
The subsequent step in task analysis is carrying out instructional research to establish the current state of knowledge and skills among first-year undergraduate students. According to a literature review on students’ perspectives and previous test responses, many students have difficulty understanding and appropriately applying calculus concepts in solving problems. They do not have adequate algebraic skills to apply these manipulations when answering open-ended questions efficiently. However, most of them have mastered calculus concepts and formulas; the issue is using them correctly.
Another essential aspect of task analysis is writing specific learning objectives that students will accomplish. These SMART goals should portray the processes and tasks students should master and their assessment procedures. At the end of this lesson, learners will be able to think critically about open-ended and word questions and apply the correct formula to solve them. They will appropriately manipulate algebraic functions to find solutions to problems and interpret graphs correctly. Lastly, learners will attain at least sixty percent in the final examinations. Developing criterion tests is the fifth step of task analysis, and it involves monitoring the effectiveness and progress of the instruction for first-year students. In this case, students will be tested through open-ended questions that represent real-life situations, true or false questions, and multiple-choice queries.
Learner’s Entry and Subordinate Skills
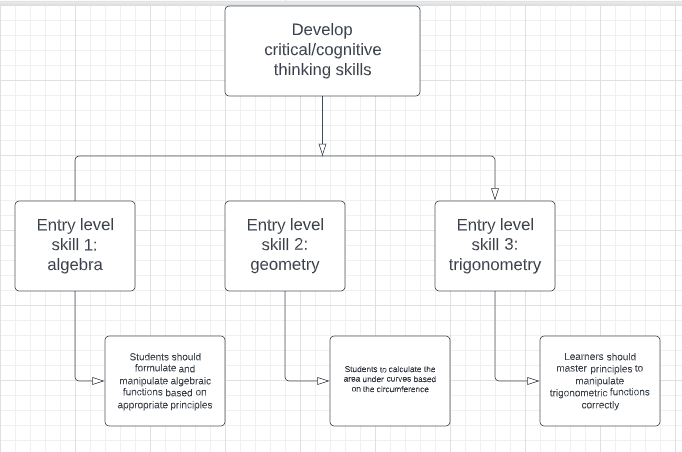
Determining learners’ entry and subordinate behaviors is another critical aspect of task analysis. Understanding students’ behaviors, motivational levels, and traits will help educators design appropriate learning techniques. The entry skills needed in an introduction to calculus lesson are understanding trigonometry, algebra, and geometry concepts. Students are required to know the principles of manipulating algebraic functions, calculate the area and volume of simple and 3D shapes, and master essential trigonometric functions, such as sin(x), cos(x), and tan(x) (Nursyahidah & Albab, 2017). Subordinate skills may include manipulating linear functions, operations of numbers, and probability and statistics knowledge. Even though learners are never ready to handle the complex aspects of calculus, they are highly motivated and have a positive attitude towards the lesson.
Foundational reasoning and understanding abilities are critical in learning calculus. However, entry-level students have severe weaknesses in the aforementioned capabilities since they do not know the conceptual background of the subject. Learners cannot answer all function word and proportional reasoning problems. Most of them cannot create meaningful formulas from quantities presented in a dynamic word problem. Additionally, students have difficulties composing two functions since they cannot correctly define the area under a circle in terms of circumference.
Additional Tasks for Learners to Accomplish
Additional tasks that learners will accomplish within the lesson include interpreting and comparing slopes of curves at different points and using sign diagrams to sketch curves derived from functions systematically. An instructional strategy that can be employed in teaching the lesson is active learning using visual representations. The design will enable students to visualize functions, thus activating their cognitive skills when applying formulas to these expressions (Jaafar & Lin, 2017). Additionally, active learning will familiarize them with various aspects of the lesson, making them understand the principles behind different questions or problems. Instructional materials used for this lesson include the textbook, graphical and open randomized exercises, CAS tools, SimCalc software, many examples, and understandable explanations. The remaining steps of analyzing this task involve creating and conducting formative and summative evaluations to assess the effectiveness of the learning initiative and obtain feedback from students. Finally, educators are encouraged to continually review their instructional design and revise according to feedback obtained and students’ performance outcomes.
Conclusion
To conclude, instructors need to plan lessons systematically by analyzing tasks to develop an effective, individualized instructional strategy. By focusing on the conceptual aspects and calculations involved in the introduction to calculus lesson, students will better understand the subject. They will sharpen their cognitive, arithmetic, and algebraic skills to ensure they apply the right concepts and formulas to the appropriate problem. As a result, significant improvement and success will be seen in their grades. Additionally, they will be well equipped to apply the acquired knowledge in subsequent calculus units.
References
Almazyad, R., & Alqarawy, M. (2020). The design of Dick and Carey Model. In Society for information technology & teacher education international conference (pp. 544-547). Association for the Advancement of Computing in Education (AACE).
Brown, A. H., & Green, T. D. (2020). The essentials of instructional design: Connecting fundamental principles with process and practice. Routledge.
Gordon, L. (2022). High calculus failure rates thwart students across CSU. EdSource. Web.
Hitt, F., & Dufour, S. (2021). Introduction to calculus through an open-ended task in the context of speed: Representations and actions by students in action. ZDM–Mathematics Education, 53(3), 635-647. Web.
Jaafar, R., & Lin, Y. (2017). Assessment for learning in the calculus classroom: A proactive approach to engage students in active learning. International Electronic Journal of Mathematics Education, 12(3), 503-520. Web.
Khoshaim, H., & Aiadi, S. (2018). Learning calculus concepts through interactive real-life examples. African Journal of Educational Studies in Mathematics and Sciences, 14, 115-124.
Nursyahidah, F., & Albab, I. (2017). Investigating student difficulties on integral calculus based on critical thinking aspects. Jurnal Riset Pendidikan Matematika, 4(2), 211-218. Web.
