Introduction and Rationale
This curriculum is designed for sixth-grade students learning mathematics as part of their public education. The curriculum focuses on developing students’ abstract mathematical thinking skills related to the ability to transfer and effectively use problem-solving procedures in real life. Mathematics education is essential for the modern individual to systematize, structure, and categorize knowledge (Wile, 2018). Accordingly, learning to extrapolate mathematical experiences into real life allows students to develop critical thinking and abstraction skills and demonstrate increased engagement with the subject matter. The program includes seven lessons based on the CCSS standards, making this plan regarded as academically robust and scientifically sound (CDE, 2021). This report offers a detailed outline of the lessons, materials, and resources used during the course.
Instructional Materials and Assessment
Lesson #1 — Introduction to the Unit Structure/Repetition of Arithmetic Operations in Life Situations
The teacher introduces the topic of the current unit and discusses the importance of applying mathematical skills to real-life situations. To be convincing, the teacher uses examples demonstrating how a practical life without mathematics is impossible: shopping for goods, fixing the house, and predicting the weather. For this purpose, the teacher shows the students a hand-crafted presentation in which the examples described by the teacher are confirmed visually to address both sixth-grade students’ perceptual channels (Mukhtoraliyevna & Madaminkhonqizi, 2022). After the introductory part, the teacher proceeds to the immediate topic of this lesson, which includes a reiteration of previously taught knowledge of arithmetic counting.
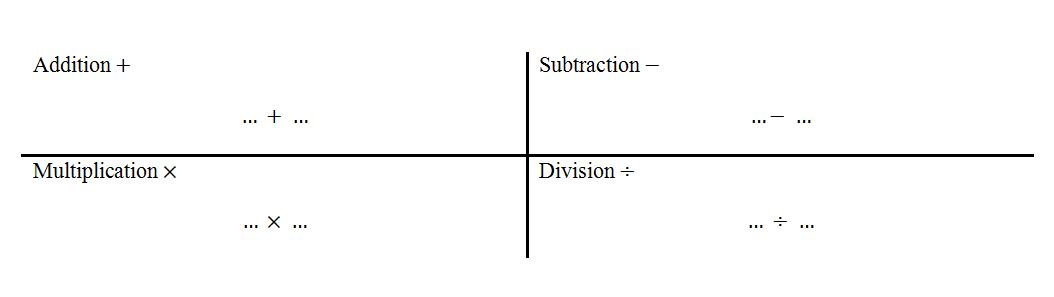
The teacher begins with an open-ended question to the class in which he clarifies with the students what counting procedures (addition, subtraction, multiplication, and division) they remember and which ones they find most challenging. Following the students’ dictation, the teacher divides the board space into four parts and prints the names of the procedures. Next to this, the teacher writes mathematical symbols (+, -, ×, ÷) and asks any of the students to come to the board and sort these symbols according to the procedure; the class checks this student’s result. The teacher then asks specific students to name random numbers and writes the named numbers on the board in the appropriate areas. This creates four problems for each specific arithmetic procedure, an example shown in Table 1.
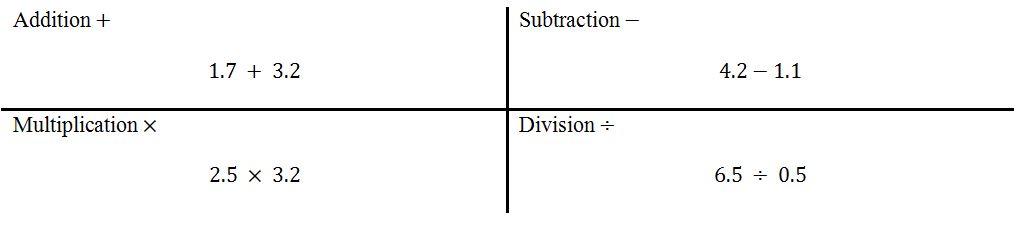
After the students have remembered the procedures for arithmetic counting, the teacher reminds them that in addition to integer operations, arithmetic also works with decimals. The teacher writes down decimal numbers (Table 2) to show the procedures for calculating with them. After the repetition, the teacher calls four new students to the board one at a time and asks them to produce their example and tell it to an abstract five-year-old in a way that the child can understand. The teacher hands the students individual task cards (Figure 1) in which they must solve the examples given. Finally, the student is asked to complete a self-assessment card (Figure 2) to assess their knowledge of the repeated topic (ODE, 2021). As a homework assignment, the teacher asks the children to survey their relatives to select 16 random numbers and then record appropriate arithmetic procedures with them.

Lesson 2 — Solving Practical Problems Based on Arithmetic Calculations
As part of the second lesson, the teacher collects the children’s homework and checks it later. After reflecting on the first lesson, the teacher offers students several life problems using arithmetic procedures. Specific tasks include speed, repair, timing, and age; some examples of these tasks are shown in Figure 3. After several examples have been solved on the board, the teacher suggests that some students go to the board and solve additional problems, after which the whole class openly discusses the correct solution and alternatives. Twenty-five minutes before the end of the lesson, the teacher distributes individual problem cards to the students so that they can practice solving.

Lesson 3 — Working with Diagrams and Charts
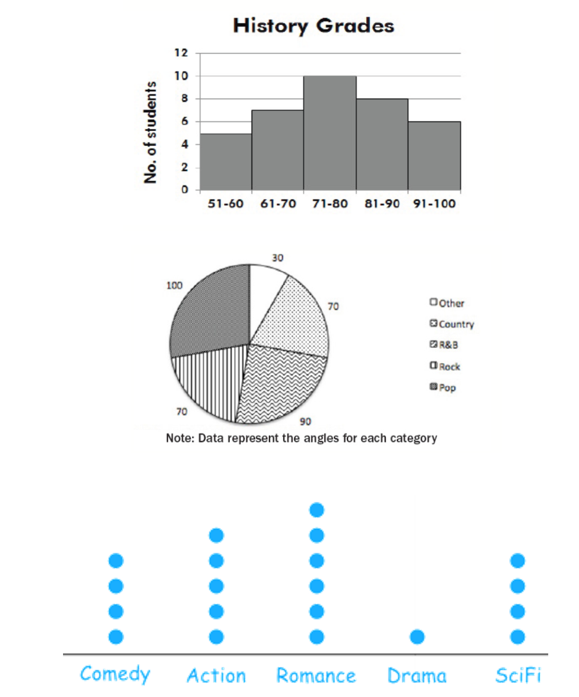
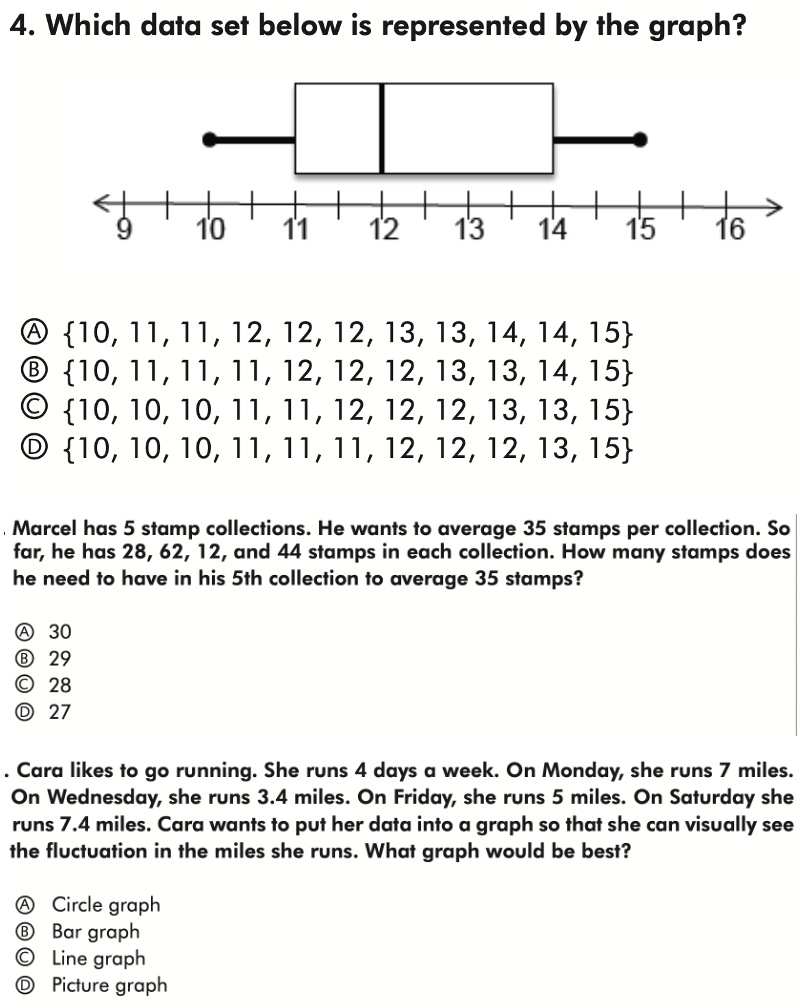
At the beginning of the lesson, the teacher plays a short video clip (https://www.youtube.com/watch?v=o43F47OkCys) to the children and, after watching it, asks them what forms of information recording they saw. The teacher starts an open discussion and asks the students to produce different connections between the data and to think about what visualization format would be most convenient for them (each student is interviewed). After the discussion, the teacher turns on the previously collected visualizations (Figure 4), asks the students what variables they are exploring a relationship between, and asks them to describe that relationship, if possible.
The teacher gives the children individual cards with statistics assignments (Figure 5) and gives them fifteen minutes to complete the card. At the end of the lesson, the teacher hands out the self-assessment cards (Figure 2) and asks each student to take turns asking a question for the whole class to understand and choose a classmate to answer. As homework, the student should experiment and choose a pair of variables for which to write down real values and construct visualizations.
Lesson #4 — Solving Practical Problems Involving Visualizations
Students present their homework projects (HW #2) and ask questions. The teacher asks each student to come to the board and solve an individual problem, and then the class evaluates the correctness of the solution.
Lesson #5 — Basics of Geometric Counting Using Figures
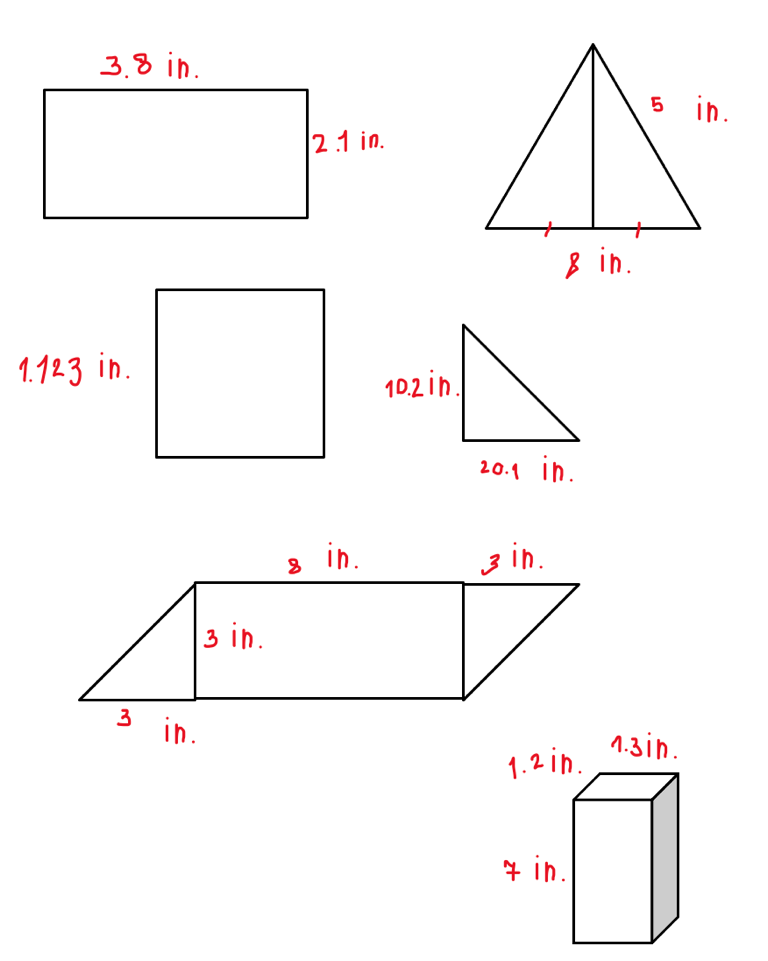
The teacher asks the children to name aloud the geometric figures they remember and then randomly pulls out the name of the student who should be told how to find the area of that figure from a basket. The teacher writes all the formulas under dictation on the board. The teacher turns on a video to the children (https://www.youtube.com/watch?v=Ybcawah5Ebk), then turns on a presentation (Figure 6) that shows different shapes and their measurements for the students to calculate the areas of each shape in their notebooks individually. The teacher asks the students on the blackboard to explain the problem-solving mechanism to a five-year-old. The students fill out self-assessment cards (Figure 2). As homework, the teacher asks the children to sketch in their notebooks some of the real-world objects that have recognizable geometric shapes (TV, table, paper airplane).
Lesson #6 — Solving Problems to Calculate Volume, Perimeter, and Area
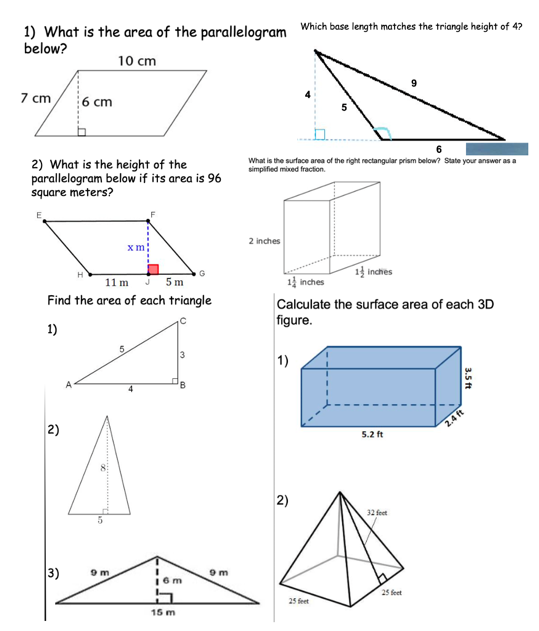
An individual lesson in which children complete geometric problem-solving tests (Figure 7). In the end, they turn in their worksheets and discuss how complex the problems were for them.
Lesson #7 — Competitive Game on All Topics
The teacher divides students into teams of three, each of which should have an advanced and a lagging student. The teacher gives each team individual task cards (Fig. 1, Fig. 3, Fig. 5, Fig. 7) and gives them a few minutes to find solutions. Afterward, the teacher shows all the teams the expected speed tasks and records which team solved the tasks faster and more correctly. At the end of the lesson, the teams present their solutions, after which each team scores points on the correctness of the solution, the confidence of the presentation, and the cleanliness of the drawings. The teacher tallies up the points and summarizes the results, assigning grades.
Lesson Plan #1&2
- Subject: Math
- Lesson Plan Title: Introduction to the Unit Structure/Repetition of Arithmetic Operations in Life Situations/ Solving Practical Problems Based on Arithmetic Calculations
- Time Frame: 60 min. (2x)
- Learner Level: Sixth grade, intermediate level
- Standards Assessed: 6.NS.A.1, 6.NS.B.2, 6.NS.B.3, 6.NS.B.4, 6.NS.C.8
- Objective: Recall the basic procedures of classical arithmetic/Reinforce understanding of arithmetic counting by practicing problem solving.
Lesson Plan #3&4
- Subject: Math
- Lesson Plan Title: Working with diagrams and charts/Solving practical problems involving visualizations
- Time Frame: 60 min. (2x)
- Learner Level: Sixth grade, intermediate level
- Standards Assessed:.RP.A.2, 6.RP.A.3, 6.RP.A.3C
- Objective: Recall the differences between diverse ways of visualizing information, namely graphs, bar charts, pie charts, and tables/Transfer experience with visualizations to specific tasks.
Lesson Plan #5&6
- Subject: Math
- Lesson Plan Title: Basics of geometric counting using figures/Solving problems to calculate volume, perimeter, and area
- Time Frame: 60 min. (2x)
- Learner Level: Sixth grade, intermediate level
- Standards Assessed: 6.G.A.1, 6.G.A.2, 6.G.A.4
- Objective: Remember the traditional flat geometric shapes, such as triangles, prisms, rectangles, and squares. Play a competitive game in groups to incorporate the knowledge from the six lectures.
Lesson Plan #7
- Subject: Math
- Lesson Plan Title: Competitive game on all topics
- Time Frame: 60 min.
- Learner Level: Sixth grade, intermediate level
- Standards Assessed: All of the previously listed
- Objective: Integrate the information learned in six lessons by playing a competitive game in teams.
References
CDE. (2021). What are the Common Core Standards? California Department of Education. Web.
Doria, S. (2021). Plane & solid figures | grade 6. YouTube. Web.
Khan Academy. (2021). 6th Grade math mini-lessons aligned with Khan Academy skills for an entire year! [PDF document]. Web.
Lumos Skill Builder. (2020). Grade 6 statistics and probability freebie [PDF document]. Web.
Mukhtoraliyevna, Z. S., & Madaminkhonqizi, S. M. (2022). Methods of mnemonics in pedagogical work with elementary school students. International Journal of Culture and Modernity, 13, 44-52.
ODE. (2021) Teaching students self-assessment [PDF document]. Web.
Ong, A. (2020). Tables and bar graphs. YouTube. Web.
Wile, E. (2018). Why is math so important for kids to learn? Hello Motherhood. Web.
